Here , is the mathematical way of finding the rolling radius of tire:-
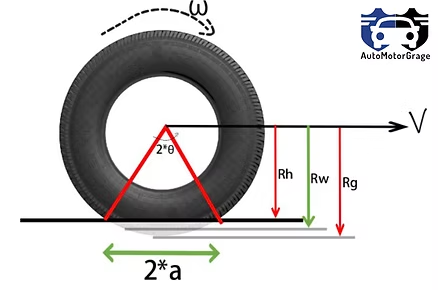
In this figure we have,
Rg – Geometric radius of tire
Rh – loaded height of tire
Rw – effective/Rolling radius
2*a – length of contact patch
2*θ – Tire print angle
ω – Angular velocity
V – wheel forward velocity
Note :- Rolling radius is somewhere between loaded height and the geometric radius.
The tire vertical deflection is:-
Rh=Rg*cosθ
a =RgSinθ (1)
Rg-Rh = Rg (1-cosθ) (2)
If the motion of the tire is compared to the rolling of a rigid disk with radius Rw, then the tire must move a distance,
a = Rw * θ (3)
From equation (1) & (3) we get ,
Rw = Rg* Sinθ/θ
Expanding Sinθ/θ ,using taylor series ,
Sinθ = θ – (1/6)*θ^3
Taking only 2 terms from taylor series,
Rw=Rg*( 1 – (1/6)*θ^2 ) (4)
For Cosθ , taylor series can be written as,
Cosθ = 1 – (θ^2)/2
θ^2 = 2*(1- Cosθ )
From equation (2) we can write,
θ^2 = 2*(1- Rh/Rg) (5)
Putting (5) in equation (4) we get,
Rw = Rg*[ 1 – 1/3*(1-Rh/Rg) ]
(Above equation is the value of rolling radius).
Rh is a function of tire load Fz,
Rh = Rg – (Fz/kz)
Where,
kz is the vertical stiffness.